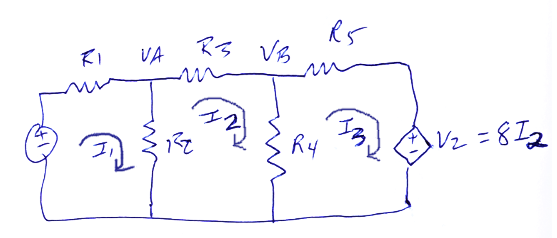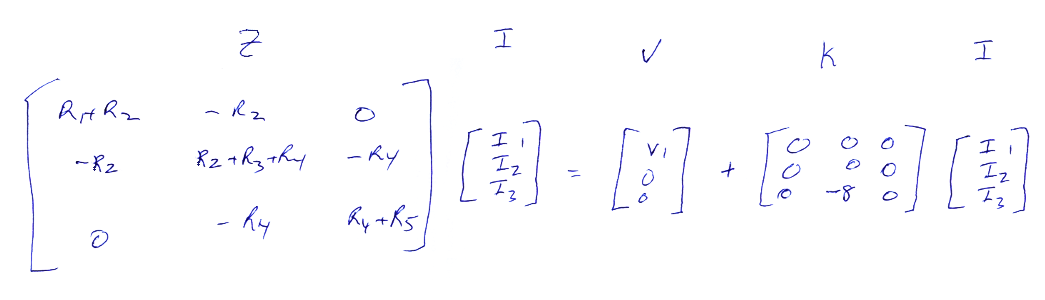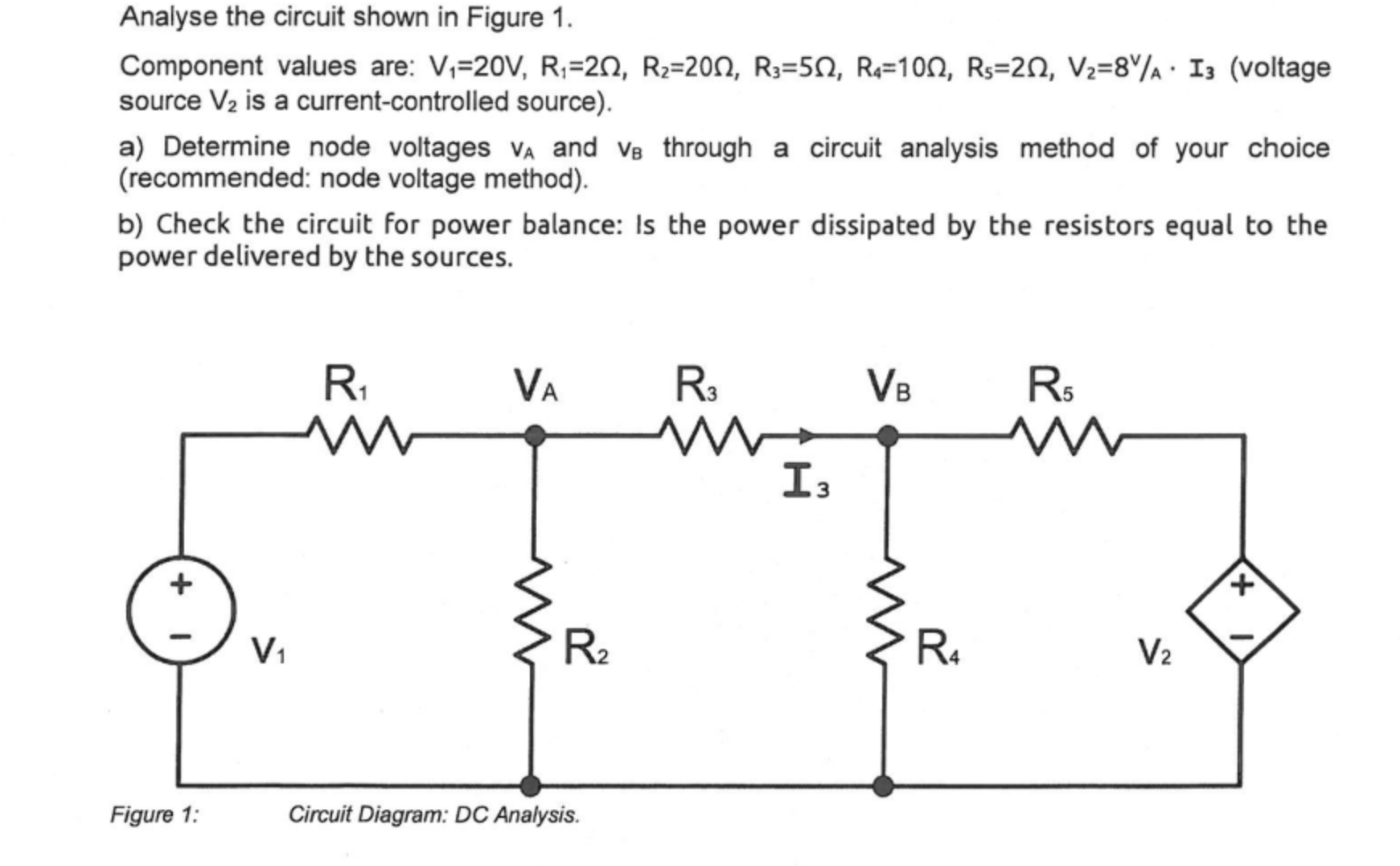
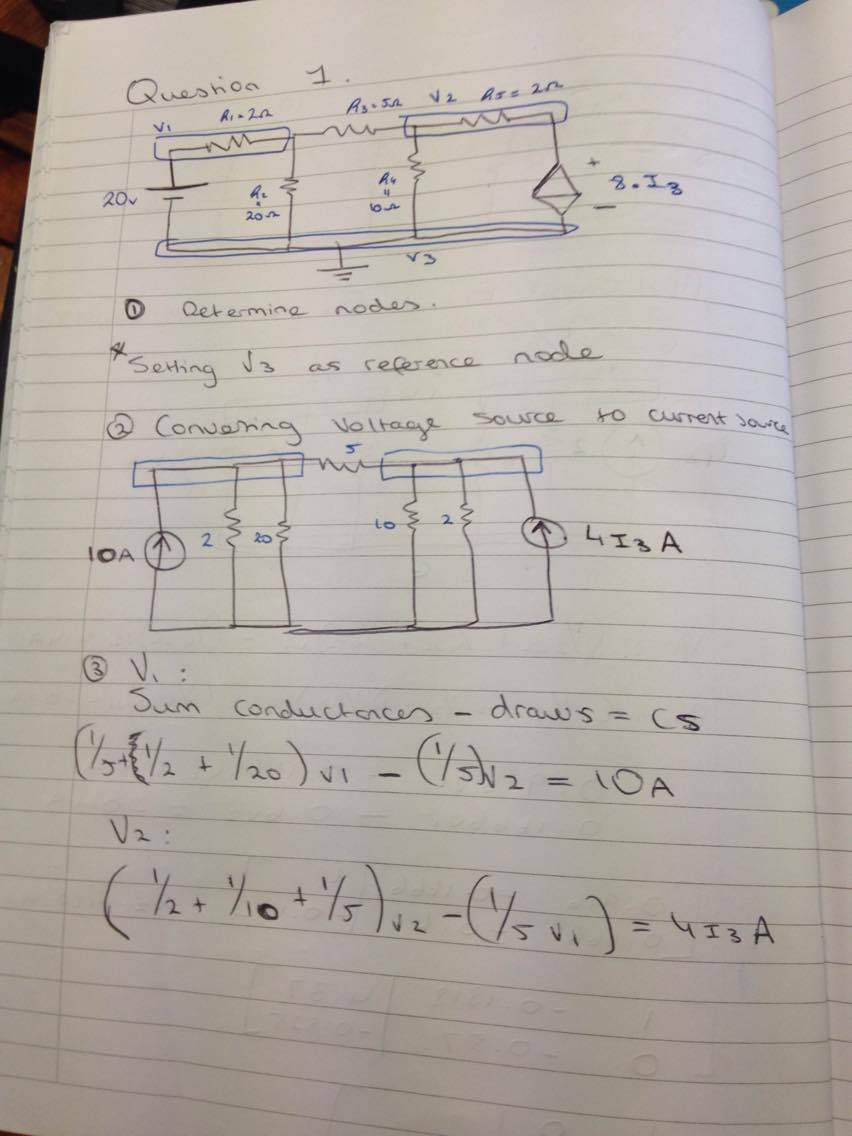
 I am carrying out node voltage analysis on a circuit that has 2 voltage sources.
I am carrying out node voltage analysis on a circuit that has 2 voltage sources.
- One source is independent
- The other is dependent on a current through a resistor in the circuit
After i carry out my analysis i have equations for the unknown node voltages however one equation contains the dependent source as per KCL.
Question
In essence i need to know what i am missing in my analysis,
- should the current be found first through superposition?
all help is greatly appreciated.