KVL and KCL in MOSFET small-signal model, how?

Question

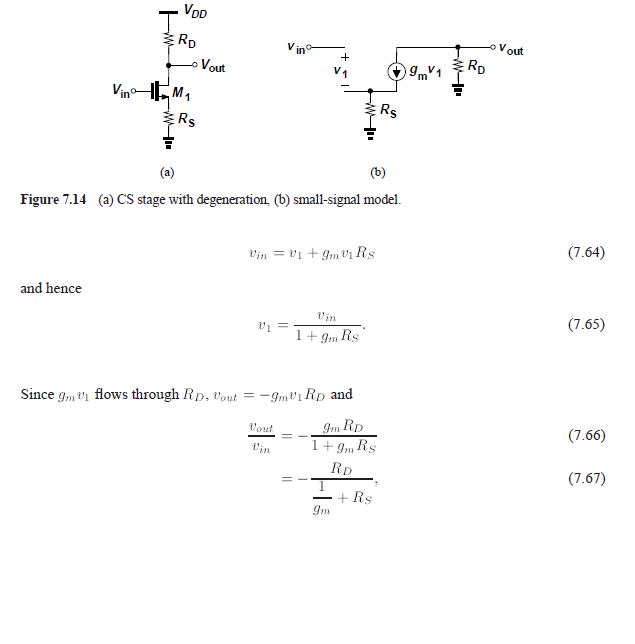
I want to find the gain in this MOSFET small-signal model.

KVL in loop 1:

\$+V_{in}-V_{gs}-g_mV_{gs}R_s=0 \iff V_{in}=V_{gs}(1+g_mR_s)\$

KVL in loop 2:

\$+V_{out}-g_mV_{gs}R_s=0 \iff V_{out}=g_mV_{gs}R_s\$

Questions:

In the answer \$V_{out}\$ should have a negative sign, \$V_{out}=-g_mR_s \cdot V_{in}\$. What have I missed?

The final answer is \$V_{out} / V_{in}=-g_mR_d/(1+g_mR_s)\$. (*)

How can the current through \$R_d\$ be \$g_mV_{gs}\$? Shouln't there instead be a KCL in \$V_a\$, so the current through \$R_d\$ is \$V_{out}/R_d\$?

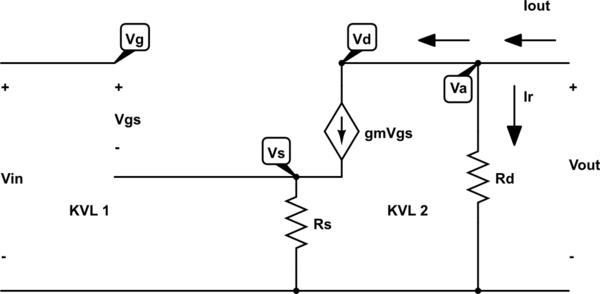
^{simulate this circuit – Schematic created using CircuitLab}

Update with KCL in \$V_a\$:

\$I_{out}-i_d-I_r=0\$

where \$i_d=g_mV_{gs}\$ and \$I_r=V_{out}/R_d\$, so

\$I_{out}=g_mV_{gs}+V_{out}/R_d\$.

I'm stuck here, how can I find equation (*) above?

Answer 1

KVL in loop 2 is missing the voltage across the current source. Because that's an unknown, instead combine your KCL expression with your KVL loop 1 expression:

$$ V_{in} = V_{gs}(1+g_mR_s) \\ I_{out} = g_mV_{gs}+V_{out}/R_d \implies V_{out} = (I_{out} - g_mV_{gs})R_d \\ \therefore \frac{V_{out}}{V_{in}}=\frac{I_{out}R_d - g_mV_{gs}R_d}{V_{gs}(1+g_mR_s)}=\frac{I_{out}R_d/V_{gs} - g_mR_d}{1+g_mR_s} $$

Now if and only if \$I_{out} = 0\$, the expression reduces to:

$$ \frac{V_{out}}{V_{in}}=\frac{-g_mR_d}{1+g_mR_s} $$

which is the result you're after.

Answer 2

Donsert you can find the solution in the following figure