Matching network and filter design

Question

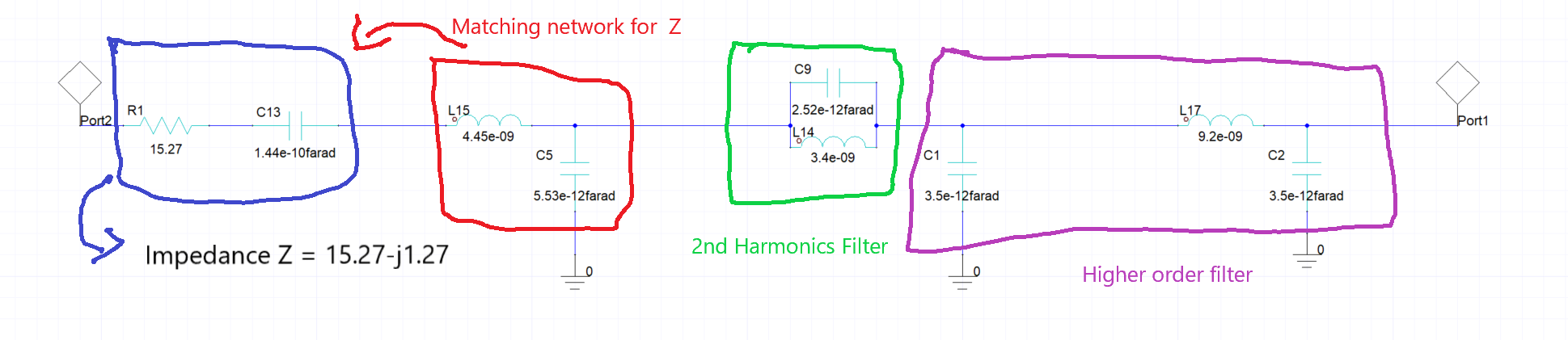
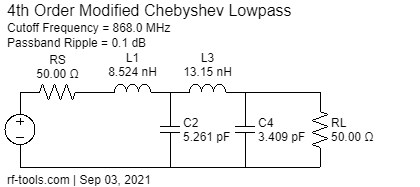
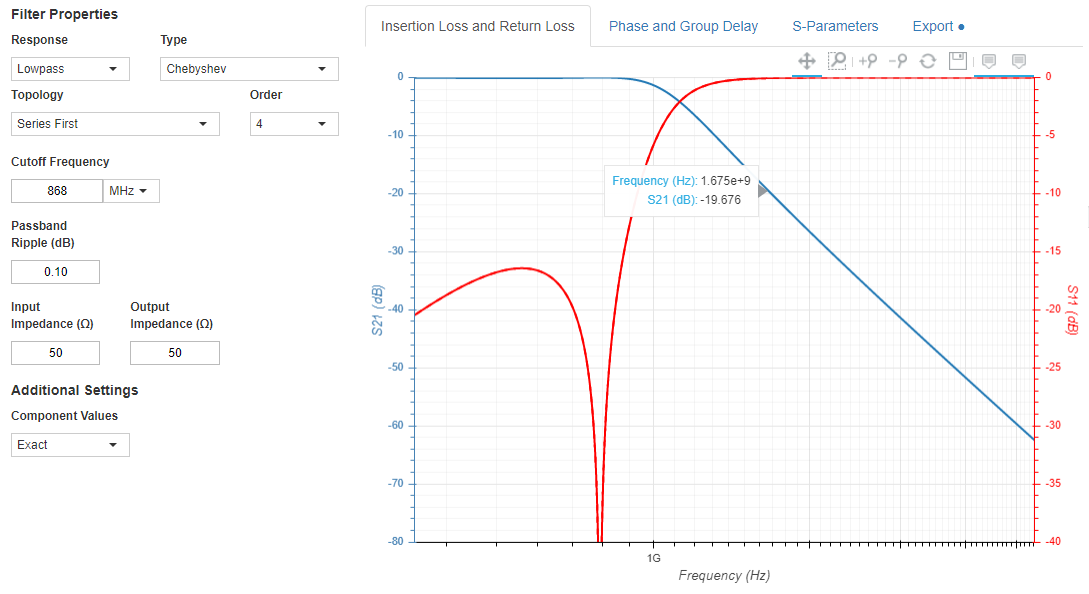
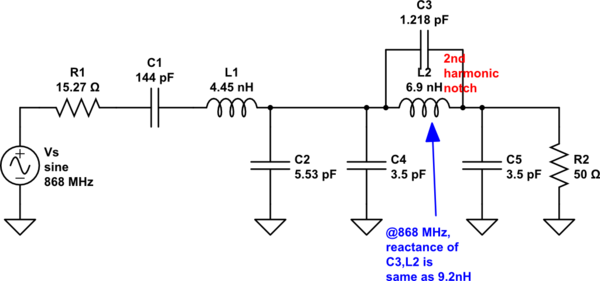
I am designing matching network at 868MHz frequency. I have a source impedance of Z=15.27-j1.27. In order to match that with 50 Ohm, I implemented LC matching network (red color). After that in order to filter second harmonics, I implemented band stop filter (green color). Following that, in order to filter higher order, I implemented CLC filter (purple color). All these are designed for 868MHz frequency.

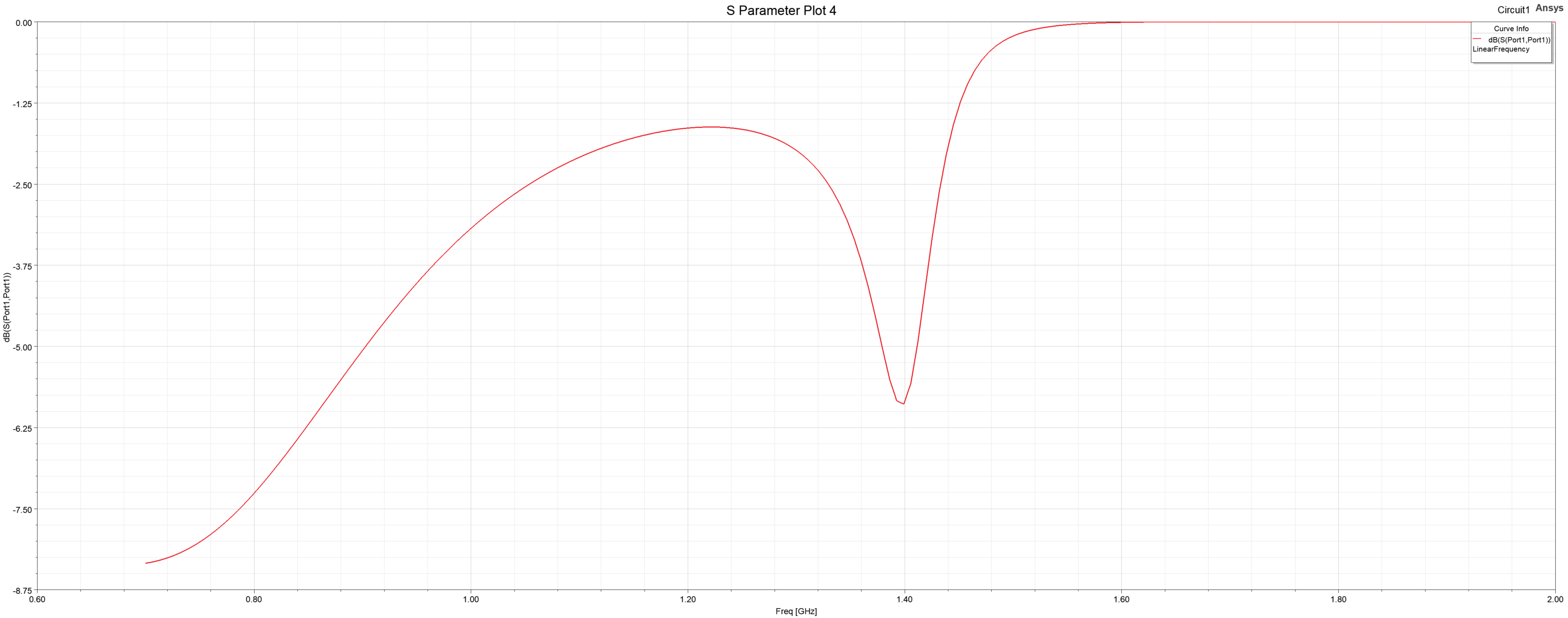
But my problem now is that, when I implemented and analyzed this circuit in Ansys Circuit, I am not getting required output. I have attached my circuit and its result below. Please help me to solve this and correct if there are any mistakes in the component values at the required frequency 868MHz.

Answer 1

Please help me to solve this and correct if there are any mistakes in the component values at the required frequency 868MHz.

Your 2nd harmonic filter looks likely to be the culprit. I can see that your final stage pi-filter is fine.

My calculator indicates 9.168 nH and 3.6673 pF for the inductor and capacitors in that for 868 MHz with 50 Ω matching but, I suspect that your 2nd harmonic filter is not producing anything like a 50 Ω input and output impedance at 868 MHz and this will ruin the performance of the pi-filter.

Your Z matching network also looks about right (using my calculator) for converting 15 Ω input to 50 Ω .

Concentrate on the band stop filter is my advice and, maybe think about making your final pi-filter cascaded with another to give more high frequency roll-off - see further down the 1st linked page (or just increase the Q of the pi-filter as per changing \$R_X\$ as indicated. Then you can get rid of the band-stop filter quite possibly.

Maybe try this Cauer/Chebyshev filter for the bandstop or the pi and hit both in one go: -

There's about 20 dB attenuation at 1736 MHz.

Answer 2

Inserting that 2nd-harmonic filter between matching section, and low-pass section is improper design. This network looks like an inductor at the design frequency of 868Mhz.

However, if you wish to knock down 2nd harmonic specifically, you can do so by modifying the low-pass filter's inductor to include a parallel capacitor. Doing this will impede the low-pass filter's attenuation in its stop-band (above 868 MHz). So expect that 3rd, 4th, 5th...harmonics will be more visible.
Your inductor L9, currently at 9.2 nH is reduced in value and a small capacitor is added in parallel. At 868 MHz, the parallel combination should be 50.17 ohms (inductive), while at 1736 MHz, the parallel combination is infinite-Z. You may find that component tolerances become tight. The filter network now becomes something like this:

^{simulate this circuit – Schematic created using CircuitLab}

If the higher-order harmonics have risen to objectionable levels, add another 50-ohm low-pass filter section to this filter's output. It is possible that adding another section may knock down 2nd harmonic enough that C3//L2 (above) could revert back to the original 9.2 nH single inductor.