Propagation delay from a graph

Question

I'm in the edX class and failed on all my three attempts on one of the questions. Clearly my reasoning is wrong, but I can't figure out where exactly. I would really appreciate if somebody could help me answer it.

Here is the figure for the question:

Here are the questions:

1. What is the contamination delay?
2. What is the propagation delay?

Horizontal resolution is 20ps.

As I said before, I've failed on all 3 attempts. But I sill would like to know how to solve this. Any help is appreciated.

Answer 1

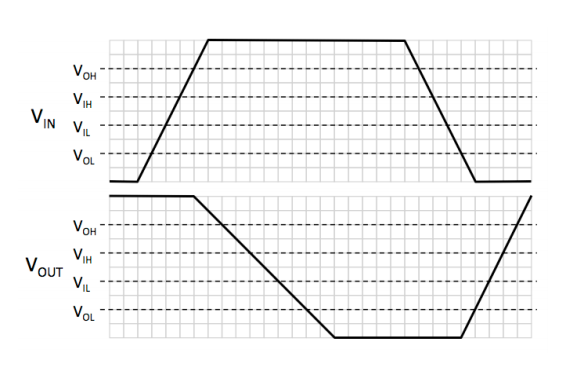
"Contamination delay" seems to be a new term for what we old-timers used to call "minimum propagation delay". It's the minimum time from when the input crosses its first threshold to when the output crosses its first threshold.

Similarly, "propagation delay" is what I would call "maximum propagation delay". This would be the time from when the input crosses its final threshold until the output crosses its final threshold.

There may be different numbers for high-to-low and low-to-high transitions.

I'm not sure why your diagram shows different threshold levels for inputs vs. outputs. Perhaps that's intended to account for noise margins within the circuitry.

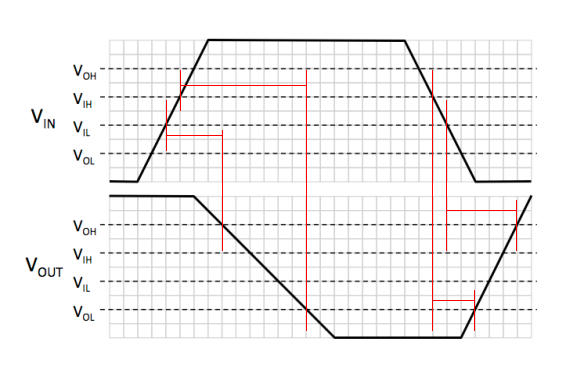
For the first transition, with the output going high-to-low:

• t_PHL(MIN) = 80 ps (contamination delay)
• t_PHL(MAX) = 180 ps (propagation delay)

For the second transition, with the output going low-to-high:

• t_PLH(MIN) = 60 ps (contamination delay)
• t_PLH(MAX) = 100 ps (propagation delay)

When accounting for propagation delays through a chain of logic, you have to assume that any given node is "undefined" between the two delay values for a given transition.