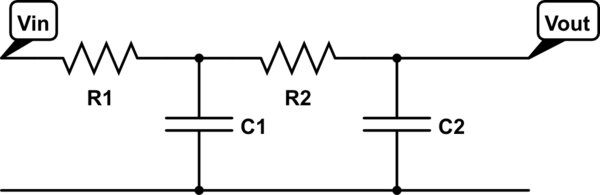
I came across with the term "degenerate circuit" when I was studying solving linear differential equations with Laplace transform. I have learned that a system is called degenerate when the determinant of coefficient matrix (characteristic polynomial) is zero.
I've searched a lot to try to understand the concept of "degenerate circuit" but I could not fully understand it. Some fragments of what I have searched:
Network Theory and Filter Design (Vasudev K. Aatre)
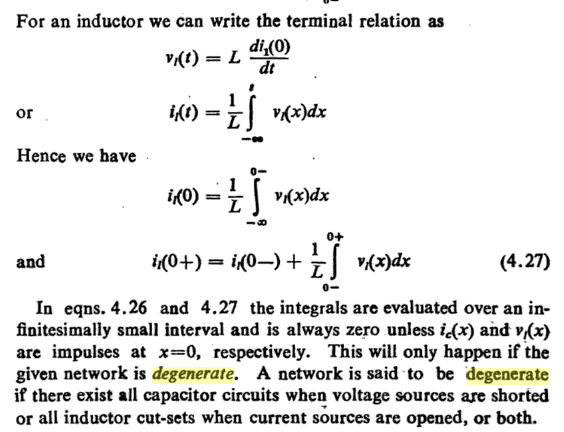
Electric Circuits And Networks (For Gtu) (Kumar K. S. Suresh)

Linear Circuit Transfer Functions: An Introduction to Fast Analytical Techniques (Christophe P. Basso)
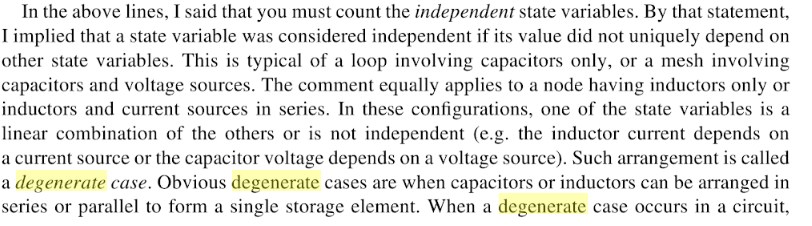
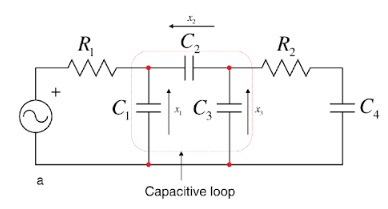
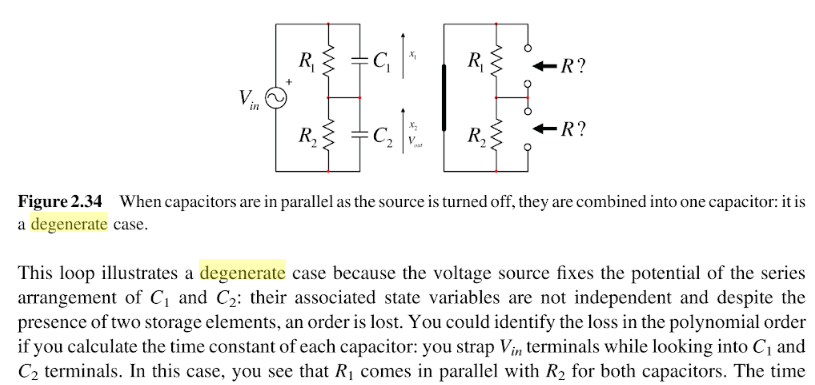
My questions are:
- What is the definition of "degenerate circuit"? (I think Basso's book 1st fragment answered it, but I didn't understand)
- What are the theoretical implications of a circuit being degenerate, in terms of stability?
- In "real life", what does it mean to be degenerate? A degenerate circuit, if built, would not work?