Does collector feedback bias work like this in real life?

Question

I am reading about collector feedback biasing in BJT transistors.

Here is how it works:

Now suppose for some reason, a temperature change perhaps, β increases. This should cause an increase in IC. An increase in IC, though, would cause an increase in the drop across RC due to Ohm's law. This, in turn, would force VC to drop.

Here is the key: VC is also equal to the drop across RB plus the voltage VBE. The base-emitter potential is fixed at approximately 0.7 volts so any decrease in VC is reflected as a decrease in voltage across RB. By Ohm's law, that means that IB must decrease by a similar proportion. This decrease tends to offset the initial tendency of the collector current to increase.

Does this circuit work sequentially in real life as above described above?

When beta increases the collector current, the base current, Vce changes instantly so I can't imagine how it follows as above.

Answer 1

Does collector feedback bias work like this in real life?

Yes it does; it creates negative feedback and, that concept is widespread in analogue electronics (particularly op-amps). Negative feedback is the "great stabilizer" of analogue circuits.

Fighting against the basic problems of a BJT circuit is something that has to be accommodated anyway (whether negative feedback is used or not).

Answer 2

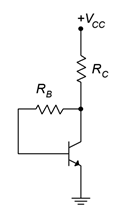
Objective

Let's say that \$I_{_\text{C}}\$ is the DC operating point value you want to evaluate for its stability against certain changes. And let's say that temperature is one of these and that device variations are another of these.

Detailing the objective

The first thing you need to do is narrow down what temperature affects and, if possible, how it affects those things. Temperature will impact both \$\beta\$ and also \$V_{_\text{BE}}\$.

There are complex equations, depending upon something called the Boltzmann factor, that impact the saturation current model parameter, which itself impacts \$V_{_\text{BE}}\$. The range seems to be about from \$-1.8\:\text{m}\:\frac{\text{V}}{^\circ\text{C}}\$ to about \$-2.4\:\text{m}\:\frac{\text{V}}{^\circ\text{C}}\$ But in general, we can say that \$V_{_\text{BE}}\$ varies by about \$-2\:\text{m}\:\frac{\text{V}}{^\circ \text{C}}\$ near room temps. This works out to about a \$- 1\% \$ change for each \$+ 1\% \$ change in temperature.

\$\beta\$ will be a different thing. It increases with increases in temperature. But let's just assume for now that \$\beta\$ changes by \$+ 1\% \$ for each \$+ 1\% \$ change in temperature.

The above doesn't account for changes in the Early Effect and a variety of other impacts. But we can assume those away, for now.

Capturing the boundaries

So, for a \$\pm 30\:^\circ\text{C}\$ temperature range we can expect to see \$V_{_\text{BE}}\$ varies about \$\pm 10\% \$, but inversely so, and \$\beta\$ varies also about \$\pm 10\% \$, but in the same direction.

As regards device variations, let's say that \$V_{_\text{BE}}\$ varies about \$\pm 10\% \$ and that \$\beta\$ varies about \$\pm 50\% \$.

Let's assume all these elements are orthogonal to each other and apply RMS to them to get a \$V_{_\text{BE}}\$ variation of about \$\pm 15\% \$ and a \$\beta\$ variation of about \$\pm 50\% \$.

This gives us some magnitudes to work with for the circuit.

Quantitative circuit analysis: \$I_{_\text{C}}\$

Oh. Almost forgot. What about the circuit? I guess we need some formulas!

If you analyze your circuit using KCL and KVL you will find that:

$$I_{_\text{C}}=\beta\cdot\frac{V_{_\text{CC}}-V_{_\text{BE}}}{R_{_\text{B}}+\left(\beta+1\right)R_{_\text{C}}}$$

To see how, read the following SymPy/Python code:

var( 'rb rc vb vc ib ic beta ie vbe vcc' )
eq1 = Eq( vc/rc + vc/rb + ic, vb/rb + vcc/rc )    # KCL collector node
eq2 = Eq( vb/rb + ib, vc/rb )                     # KCL base node
eq3 = Eq( vcc - rc*(ic+ib) - rb*ib - vbe, 0 )     # KVL through resistors to ground
eq4 = Eq( ic, beta*ib )                           # BJT
eq5 = Eq( ie, ic + ib )                           # BJT
ans = solve( [ eq1, eq2, eq3, eq4, eq5 ], [ vc, vb, ic, ib, ie ] )

ans[ic]
-beta*(vbe - vcc)/(beta*rc + rb + rc)

pprint( ans[ic] )
-β⋅(vbe - vcc)
───────────────
 β⋅rc + rb + rc

Sensitivity

A sensitivity equation is used to relate the %-change of one thing to the %-change of another thing. This looks like: \$\frac{\frac{\text{d}\, y}{y}}{\frac{\text{d}\, x}{x}}=\frac{\text{d}\, y}{\text{d}\, x}\cdot\frac{x}{y}\$, which gives you the %-change of y as compared to the %-change of x.

In this case, I find:

$$\begin{align*} \left|\%\,I_{_\text{C}}\right|&=\%\,V_{_\text{BE}}\cdot\frac{V_{_\text{BE}}}{V_{_\text{CC}}-V_{_\text{BE}}} \\\\ \left|\%\,I_{_\text{C}}\right|&=\%\,\beta\cdot\frac{R_{_\text{B}}+R_{_\text{C}}}{R_{_\text{B}}+\left(\beta+1\right)R_{_\text{C}}} \end{align*}$$

The SymPy code looks like this:

simplify( derivative( ans[ic], vbe ) * vbe / ans[ic] )
vbe/(vbe - vcc)

simplify( derivative( ans[ic], beta ) * beta / ans[ic] )
(rb + rc)/(beta*rc + rb + rc)

Getting more specific

So, let's assume \$I_{_\text{C}}=2\:\text{mA}\$ and \$V_{_\text{CC}}=10\:\text{V}\$. Assuming also a design value of \$\beta=200\$ and \$V_{_\text{BE}}=700\:\text{mV}\$ and a quiescent collector operating point near \$3.4\:\text{V}\$, we might then specify \$R_{_\text{C}}=3.3\:\text{k}\Omega\$ and \$R_{_\text{B}}=270\:\text{k}\Omega\$. (Recomputing, we'd then find \$I_{_\text{C}}=1.99\:\text{mA}\$. Close enough.)

From these values and the above %-change estimates, we'd say that the collector current might change by about \$\pm\,14.6\%\$ over part and temperature variations of \$\beta\$ and by about \$\pm\,1.1\%\$ over part and temperature variations of \$V_{_\text{BE}}\$.

The SymPy code looks like:

abs( simplify( derivative( ans[ic], beta ) * beta / ans[ic] ).subs(
    { beta:200, rb:270e3, rc:3.3e3 }
) * 50 )
14.6415943426551

abs( simplify( derivative( ans[ic], vbe ) * vbe / ans[ic] ).subs(
    { beta:200, rb:270e3, rc:3.3e3, vcc:10, vbe:.7 }
) * 15 )
1.12903225806452

If the argument so far has been soundly reasoned, then it's now obvious that \$\beta\$ variation swamps out \$V_{_\text{BE}}\$ variation and you should figure that there will be as much as \$\pm\,15\:\%\$ variation in the operating point over temperature and device variations.

(You could remove the orthogonality assumption I made and argue for still worse. But this gives an idea about an approach to quantify variations you care about.)

This particular circuit topology

This circuit topology is never used in isolation where a specific voltage gain is required, because the voltage gain is highly dependent upon the collector current and because it is also highly dependent upon the signal, itself. So this is used to get the highest gain possible from a BJT, with the expectation of very high distortion. It is therefore always wrapped with negative feedback by placing it within a larger circuit context to remove distortion and set the desired voltage gain.

The analysis so far tells us something useful about this topology.

To get the highest possible voltage gain from this circuit, \$R_{_\text{C}}\$ should be as large as possible without forcing the BJT into deep saturation. But we must allow for collector current variation of about \$15\,\%\$. We might have been tempted to set \$R_{_\text{C}}=3.9\:\text{k}\Omega\$ to get better voltage gain. But then we'd find a quiescent collector voltage that could be under one volt. And that's without any signal applied! So, with signal, we'd almost certainly be driving the BJT well into deep saturation. That's why this kind of analysis can be vital in deciding how much to compromise on the voltage gain in order to deal with circuit variations, even with NFB applied.

Engineering logbooks

As an aside, what you read above is something that should be placed into the engineering logbook you keep as a circuit designer. (A must-do, if you are a professional, and should-do, otherwise.) These kinds of details are important to record and to be able to refer back to, should circumstances require it, in order to show why you made the choices you made at the time. For professionals, it can save your life if things ever go to court. For professionals and hobbyists, it can save a lot of your time later on when you need to revisit a design.

In short, keep logbooks for the rest of your life!

Answer 3

The sequential reasoning you described is used quite frequently to explain the operation of many circuits, especially those in which feedback is employed.

However in the physical reality there is no such clear-cut sequence.

That sequence is an easy way of analyzing the influence of a parameter variation in a qualitative way, but that is just a simplification. With that "sequencing" you assume a certain chain of cause-effect steps whose actual justification lies in how the circuit equations works.

In particular, that reasoning is valid as long as you implicitly assume a small variation relative to a quiescent point. This is necessary because this method can be formally justified only if the circuit is linear or, as in your case, can be linearized (that small variation assumption is needed for the linearization to be performed).

This reasoning can be formally justified by writing the non-linear (maybe differential) equations system describing the circuit and applying a linearization technique. This involves things like multidimensional Taylor expansion, which in turn is based on partial derivatives and multidimensional function theory. Tough stuff for a beginner, but it is something that many electrical engineers may have to grok during their engineering university education.

However it is not something that you need to understand in order to apply that sequential method to understand how the circuit behaves, as long as you assume small variations and the circuit can be linearized around the chosen quiescent point.

Answer 4

There's a balance where the voltage at the collector Vce has just the exact value needed to feed just enough base current to maintain the collector current what's needed to get the voltage at the collector to be dropped to Vce from value Vcc. It can be coarsely calculated with Vbe and transistor's Hfe (see NOTE1). Or it can be found by simulating or by making a test circuit.

That biasing solution has one advantage: It reduces the dependency on transistor's Hfe which vary easily -50...+100% between the individuals of the same type. Also the collector leakage current doesn't affect as much the operating point as it would do in case Rb was connected between Vcc and the base.

Rb between the collector and the base essentially causes a negative feedback, which as a side effect reduces the gain in multistage transistor amplifiers. But also that can be considered as an advantage: The non-linearity of the transistor causes less distortion in a class-A amplifier stage.

NOTE1: To get the operating point (=idle state Ic and Vce) one must solve the next equation group

Ic = Hfe(Vce - 0,7V)/Rb

Vce = Vcc - Rc(1 + 1/Hfe)Ic

The first one says that Ic = Hfe * Ib

The second one says the voltage drop in Rc is the collector current plus the base current multiplied by Rc.

Answer 5

For my feeling, some comments are in order:

• The most important temperature influence on the DC bias point comes from the positive temparature sensitivity of the current Is in the well-known equation Ic=Is[exp(Vbe/Vt)-1)]
• This increase can be compensated when Vbe is externally reduced (negative DC feedback using an emitter resistor Re or Rb as shown) by a app. -2mV/K. In the latter case (Rb stabilization) it is the voltage division between Rb and the DC input resistance at the B-E junction (Rbe=Vbe/Ib) that is responsible for the Vbe reduction).
• In contrast to this sensitivity of Is, the sensitivity of the current gain B (resp.beta) is comparably small.